MATH 112 Week 9 Final Exam

MATH 112 Week 9 Final Exam | Assignment Help | Embry Riddle Aeronautical University 

1.         Evaluate.

∫x2−9x+2 dx

2.         Find the area under the given curve over the indicated interval.

y =  x²      [1, 2]

Area  =∫ x² dx     [1, 2]

3.         y =  2x³ – 24x – 2

            dy/dx  =          6x² – 24

4.         Find the equation of the curve that passes through  (2,3)if its slope is given by the following equation

dy/dx = 3x – 4

Slope at x = 2                        3*2 – 4 =    6 – 4   =  2

5.         f(x) = 2/3x     a = 2, b = 3    n = 2

            ∆x =  (3 – 2) /2 = ½  = 0.5

            So Intervals are            2, 2.5, 3

6.

 The​ x- and​ y-coordinates of a moving particle are given by the parametric equations below.

x = t(2t + 1)²

y = 5/ √5t + 5           

Find the magnitude and direction of the velocity when  t = 0.25

7.        

Antiderivative          f(x) = 9/x³+  1/8²

8.         Evaluate the given definite integral.

9.        

An open box is to be made from a square piece of cardboard whose sides are 16  inches​ long, by cutting squares of equal size from the corners and bending up the sides. Determine the size of the square that is to be cut out so that the volume may be a maximum.

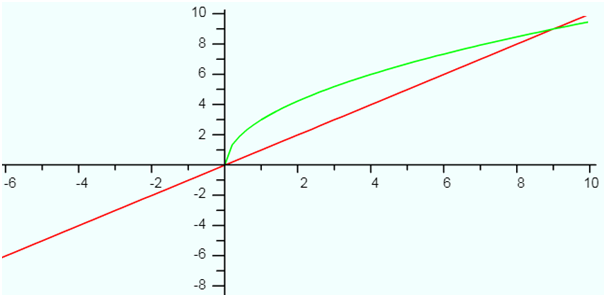
10.      

y = x

            Y = 3√x

Graph is like this

11.

A rocket follows a path given by  y =  x -  1/75 x³(distances in​ miles). If the horizontal velocity is given by

v_x =  x      find the magnitude and direction of the velocity when the rocket hits the ground​ (assume level​ terrain) if time is in minutes.

Magnitude of the velocity of the rocket at the point

Find the direction of the velocity of the rocket at the point Enter an angle θsuch that 0 degrees less than or equals theta less than 360 degrees .0°≤θ<360°.

Select the correct choice below and fill in any answer boxes in your choice.

12.

The height​ (in ft) of a flare shot upward from the ground is given by S = 89.6 t – 16t²

where t is the time​ (in s). What is the greatest height to which the flare​ goes?

Find the derivative of the function to be maximized.